Arts and Crafts in Four Dimensions

She returned the smile, then looked across the room to her youngest brother, Charles Wallace, and to their father, who were deep in concentration, bent over the model they were building of a tesseract: the square squared, and squared again: a construction of the dimension of time. It was a beautiful and complicated creation of steel wires and ball bearings and Lucite, parts of it revolving, parts of it swinging like pendulums.*

– Madeleine L'Engle, A Swiftly Tilting Planet

I wrote a couple of months ago about four-dimensional geometry. Today I'd like to continue our progress through transdimensional gulfs and sinister alien geometries by discussing the 120-cell in some detail, and also describing the workflow I used to print the three-dimensional sections and net shown below.

As usual when trying to understand the fourth dimension, it's easiest to proceed by way of analogy with lower dimensions. Imagine a two-dimensional creature, like A. Square of Flatland, existing in a planar universe. Such a creature would have an essentially one-dimensional field of vision, much as our field of vision is essentially two-dimensional (like a painting or a television screen). How would we describe a dodecahedron, that is, a polyhedron formed from twelve regular pentagons, to such a creature?

(Click to read more; I've got a lot going on in this post.)

One possibility would be to show the creature the flattened net from which the dodecahedron is folded.

The creature would see a collection of pentagons, not all of which would be visible at once, since it can only perceive the outside edges, just as you would only see the edge of a penny on a table if you knelt with your eyes on a level with the surface.

Another possibility would be to pass a dodecahedron through the two-dimensional universe as the Sphere passed before the astounded gaze of A. Square. Just as A. Square perceived the Sphere as a sequence of circular sections, our creature would perceive the dodecahedron as a sequence of polygonal sections, but the sequence would depend on whether the solid proceeded face first, edge first, or vertex first.

In each of the following animated gifs (don't you miss animated gifs?), the orange polygon represents the section cut by the two-dimensional universe. Just remember that our creature is only able to perceive the outside of the polygon, and can't take it in all in one glance as we can.

Here's the face-first passage, which proceeds from a pentagon to a decagon to an upside-down pentagon:

Now for the edge-first passage, which proceeds from a line segment to a square to a hexagon and back:

Lastly, the vertex-first passage, which proceeds from a point to a triangle to a hexagon to an upside-down triangle and so to a point again:

In this way, A. Square can conceive of the dodecahedron as being folded from a net (though in a direction he cannot visualize) or as a sequence of polygonal sections.

*

Much as the dodecahedron is formed by joining twelve pentagons along their edges, the 120-cell is formed by joining 120 dodecahedra along their faces. Each edge is shared by three dodecahedra.

Of course, three dodecahedra joined face-to-face and face-to-face around a common edge don't quite meet at their third pair of faces, but if we bend them around the edge, lifting them in a direction perpendicular to our own three dimensions, we can make them meet.

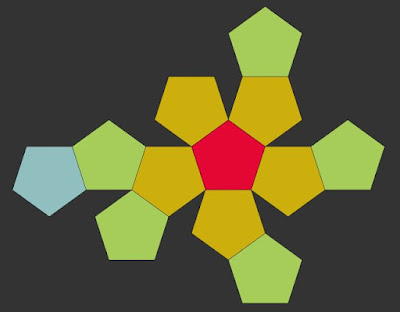
Since we are three dimensional beings, we have just as much trouble picturing such an object as our two-dimensional creature has in imagining a dodecahedron. So let's make it easy on ourselves as we did for A. Square. We could, for instance, build a net of dodecahedra.

In this virtual model (which I constructed with GeoGebra, the free educational software I use to teach) we see the "bottom" half of the net. It's sort of like the one red and five orange pentagons in our flat dodecahedral net above. But, limited to motion in three dimensions as we are, we'd have to ask an obliging fourth-dimensional creature to fold it up for us. The "bottom" cell is at the center, and invisible to our three-dimensional eyes, though our fourth-dimensional friend can see it plainly enough just by looking "down" on it. The red dodecahedra around the periphery represent the "equator" of the 120-cell. The "upper" half would be folded from an identical net, minus the equator, and fitted around this half.

*

We could also visualize the 120-cell by passing one through our three-dimensional space. If we do this in a cell-first manner, we see a sequence of polyhedra, starting with the dodecahedral cell itself. Alicia Boole Stott, whom we discussed in the previous post, built beautiful models of such sections and others. You can find pictures of them online.

I'm a visual person, and most of the math I'm interested in has to do with decorative arts, models, or constructions in one way or another. Actually, when I was in college, I switched my major from drawing-and-painting to math after seeing a geometry film in a 3D design class. (I'm also a  capricious and superficial person.) So seeing beautiful models like these motivate me to understand and to make.

I began with looking up Stott's 1900 paper, "On Certain Series of Sections of the Regular Four-Dimensional Hypersolids," and reading it.

 Actually, to be honest, I only read the first few pages, and then looked at the pictures.

The following shows partial nets for the sequence of sections of the 120-cell. Note that the shapes are all sections of the dodecahedron as shown in the animated gifs above.

These excellent diagrams have actually been used to reconstruct her models from card stock. However, I wanted to print them. Like most things in math, you pretty much have to rediscover what's known about the 120-cell in order to really understand it yourself. So I set about doing that, first by messing around on GeoGebra. I created something like a net, but with the dodecahedral symmetry of the "base" cell at the center.

Staring really hard at this construction helped me understand where Stott got her Figures VIII through XIV. One by one, I used transformations to construct the sections as concentric polyhedra. To get the scale correct, it's easiest to regard each as constructed from two-dimensional sections of a single dodecahedron the same size as the polytope's cells.

For each section (labeled VIII, IX, X, XI, XII, XIII, and XIV, after Stott), I generated a set of vertices.

Alas, GeoGebra does not at present export in the .stl format, which is what I need to print. On the other hand, 3D rendering programs don't typically lend themselves to the kind of geometric constructions we need. No doubt someone better with computers than I am would be able to write some code to bridge the gap. Here's what I did instead.

First, I put the list of vertices in the wonky GeoGebra spreadsheet, copied and pasted into Word, used the replace function to change the delimiters to tabs and returns, and copied into Excel.

Then I copied each set of vertices into Notepad using the .obj (object) file format and saved as an .obj file, which you can do, apparently.

Then I went into Blender, which I use for my print projects, and imported the .obj file, creating a set of vertices. I applied the convex hull tool and obtained the desired solid, which I then exported as an .stl file.

At long last it was time to prepare the print file. I'm using an NWA3D A31, which has a 12" x 12" bed.

Each of the sections took a few hours to print. I've discovered that the printer takes about 120% of the projected time to actually complete the job. Either the PLA is of lower quality than what I started with when we got the printer, or I've changed some setting that I shouldn't have, but the jobs come out a bit messier than I'd like, and generally require significant sanding. I suspect it's the plastic, but I'll be getting a new spool soon, so we'll see.

I next painted the sections with Folk-Art brand acrylic craft paint from Wal-Mart. I used the multi-surface stuff, which seems to adhere to the PLA pretty well. Each solid got two to three coats. I finished them with semigloss Polycrylic.

The final product is quite handsome, I think.

The seven sections are shown in order below, from the base cell on the left to the equatorial section on the right, each cut by a three-dimensional space parallel to the base cell and passing through a set of vertices. These are only half of the sections; to complete the collection, we would need to go through the first six sections again, but in reverse order, with another copy of the small dodecahedron to represent the "north pole."

I also made the partial net shown above from 75 printed dodecahedra. I had planned to do the "upper" half and connect them, but I don't have the energy right now. I'm getting ready for an art show, and it takes a long time to print, paint, finish, and glue that many dodecahedra.

And here's a top view:

I may try to repeat all of this for the 24-cell or the 600-cell in the not-so-distant future, if time allows.

*

For various reasons, I like coming up with ways of making sophisticated models using the most inexpensive of materials acquired from places like Wal-Mart, Dollar General, or Family Dollar. My 120-cell sections may have been produced on an expensive piece of equipment, but both the local public library and the junior college have printers that are available for pretty much anyone to use, and the paint and stuff all came from Wal-Mart.

Last week I made a two-dimensional model of the 24-cell (a four-dimensional figure formed from 24 octahedra) using dress pins, thread, a wooden plaque, stain, and varnish all from Wal-Mart.

The vertices are projected from four dimensional space onto the xy-plane using the coordinates described by Coxeter in Regular Polytopes. I used a single piece of string, double-covering the edges to achieve an even appearance.

I plan on making the 600-cell in the same way, but I haven't found a piece of wood the right size and shape yet.

And here's a tensegrity icosahedron built using empty ballpoint pen tubes, rubber bands, and paper clips.

The tension in the rubber bands keeps the structure in its icosahedral shape; no glue is required, and in fact the struts don't even touch each other. The idea is adapted from George Hart's instructions for the dodecahedron, but I use pen tubes instead of soda straws because they're stiffer. (One of my students came up with that. And anyway, the generic ballpoint pens they sell in big bags at Wal-Mart aren't much good for writing, so it seems appropriate to give them a better use.) The term "tensegrity" was coined by Buckminster Fuller, who used the idea in architecture.

* Quotation provided for dramatic purposes only. Ignore any mathematical or scientific implications.